No Bucks Racing Club
P.O. Box 662
Burbank, CA 91503
(C)Copyright 1991
Most autocrossers and race drivers learn early in their careers the importance of balancing a car. Learning to do it consistently and automatically is one essential part of becoming a truly good driver. While the skills for balancing a car are commonly taught in drivers' schools, the rationale behind them is not usually adequately explained. That rationale comes from simple physics. Understanding the physics of driving not only helps one be a better driver, but increases one's enjoyment of driving as well. If you know the deep reasons why you ought to do certain things you will remember the things better and move faster toward complete internalization of the skills.
Balancing a car is controlling weight transfer using throttle, brakes, and steering. This article explains the physics of weight transfer. You will often hear instructors and drivers say that applying the brakes shifts weight to the front of a car and can induce oversteer. Likewise, accelerating shifts weight to the rear, inducing understeer, and cornering shifts weight to the opposite side, unloading the inside tires. But why does weight shift during these maneuvers? How can weight shift when everything is in the car bolted in and strapped down? Briefly, the reason is that inertia acts through the center of gravity (CG) of the car, which is above the ground, but adhesive forces act at ground level through the tire contact patches. The effects of weight transfer are proportional to the height of the CG off the ground. A flatter car, one with a lower CG, handles better and quicker because weight transfer is not so drastic as it is in a high car.
The rest of this article explains how inertia and adhesive forces give rise to weight transfer through Newton's laws. The article begins with the elements and works up to some simple equations that you can use to calculate weight transfer in any car knowing only the wheelbase, the height of the CG, the static weight distribution, and the track, or distance between the tires across the car. These numbers are reported in shop manuals and most journalistic reviews of cars.
Most people remember Newton's laws from school physics. These are fundamental laws that apply to all large things in the universe, such as cars. In the context of our racing application, they are:
The first law: a car in straight-line motion at a constant speed will keep such motion until acted on by an external force. The only reason a car in neutral will not coast forever is that friction, an external force, gradually slows the car down. Friction comes from the tires on the ground and the air flowing over the car. The tendency of a car to keep moving the way it is moving is the inertia of the car, and this tendency is concentrated at the CG point.
The second law: When a force is applied to a car, the change in motion is proportional to the force divided by the mass of the car. This law is expressed by the famous equation
The third law: Every force on a car by another object, such as the ground, is matched by an equal and opposite force on the object by the car. When you apply the brakes, you cause the tires to push forward against the ground, and the ground pushes back. As long as the tires stay on the car, the ground pushing on them slows the car down.
Let us continue analyzing braking. Weight transfer during accelerating and cornering are mere variations on the theme. We won't consider subtleties such as suspension and tire deflection yet. These effects are very important, but secondary. The figure shows a car and the forces on it during a ``one g'' braking maneuver. One g means that the total braking force equals the weight of the car, say, in pounds.
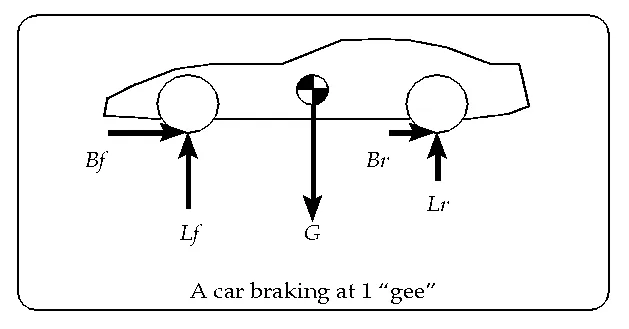
In this figure, the black and white ``pie plate'' in the center is the CG.
We don't often notice the forces that the ground exerts on objects because they are so ordinary, but they are at the essence of car dynamics. The reason is that the magnitude of these forces determine the ability of a tire to stick, and imbalances between the front and rear lift forces account for understeer and oversteer. The figure only shows forces on the car, not forces on the ground and the CG of the Earth. Newton's third law requires that these equal and opposite forces exist, but we are only concerned about how the ground and the Earth's gravity affect the car.
If the car were standing still or coasting, and its weight distribution were 50-50, then
Braking causes
The braking forces create a rotating tendency, or torque, about the CG. Imagine pulling a table cloth out from under some glasses and candelabra. These objects would have a tendency to tip or rotate over, and the tendency is greater for taller objects and is greater the harder you pull on the cloth. The rotational tendency of a car under braking is due to identical physics.
The braking torque acts in such a way as to put the car up on its nose. Since the car does not actually go up on its nose (we hope), some other forces must be counteracting that tendency, by Newton's first law.
By how much does
With the help of a little algebra, we can find out that
Thus, by braking at one g in our example car, we add 640 pounds of load to the front tires and take 640 pounds off the rears! This is very pronounced weight transfer.
By doing a similar analysis for a more general car with CG height of
These equations can be used to calculate weight transfer during acceleration by treating acceleration force as negative braking force. If you have acceleration figures in gees, say from a G-analyst or other device, just multiply them by the weight of the car to get acceleration forces (Newton's second law!). Weight transfer during cornering can be analyzed in a similar way, where the track of the car replaces the wheelbase and
Now you know why weight transfer happens. The next topic that comes to mind is the physics of tire adhesion, which explains how weight transfer can lead to understeer and oversteer conditions.
[hr]
converted by: rck@home.net
Thu Sep 29 15:11:18 PDT 1994


